Specifying line inductors for power converter noise filters

2019/02/05
Specifying line inductors for power converter noise filters Image
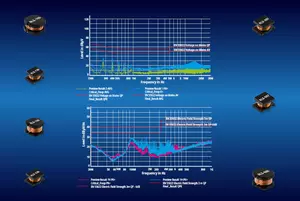
The performance over the full frequency range can vary significantly between different component suppliers for parts with the same nominal characteristics. The variation in inductor performance is examined in this whitepaper.

Table of contents

  1. Abstract
  2. Introduction
  3. LC filters help reduce output noise
  4. Parasitic effects in inductors change noise attenuation performance
  5. Input EMC filter component selection (Download/Login)
  6. RLS series (Download/Login)

Get the whole Whitepaper now

ダウンロード/ログイン

1. Abstract

Inductor–capacitor (LC) filters are commonly inserted into the inputs and outputs of switched-mode power converters to reduce reflected ripple current and output noise as well as to meet the radiation and susceptibility limits for EMC. Although the appropriate filter inductor value can be easily calculated, the inductor performance over the full frequency range can vary significantly between different component suppliers for parts with the same nominal characteristics, leading to poor results and increased conducted and radiated interference. The variation in inductor performance is examined in this whitepaper.

2. Introduction

Most modern DC power converters and all isolated DC/DC converters are of the ‘switched-mode’ type, where external DC voltages are ‘chopped’ at high frequency to produce an internal square-wave AC drive for the isolation transformer. The transformer output is then rectified back to DC, with high efficiency and low losses. A disadvantage of using switch-mode topologies is that this switching process generates high frequency ripple on the input and output pins along with conducted and radiated noise spikes that can disrupt other equipment. There is a trend for power converters to operate at ever-higher frequencies with faster slew rates to increase efficiency, but the resulting noise spectrum is much broader.

3. LC filters help reduce output noise

Any commercial power converter will have minimal filtering internally to reduce ripple and noise to a typical peak-to-peak value of about 1% of the DC output. This is acceptable in most cases, but if lower levels are required for a sensitive application, a simple solution would be to add an external LC filter (Figure 1).

The inductor impedance is theoretically zero at DC and the capacitor impedance is infinite, so the desired DC is unaffected. However, as frequency increases, inductor impedance ZL increases and capacitor impedance ZC reduces, producing an increasing ‘voltage divider’ effect.

Fig. 1: External LC filters reduce output ripple and noise
The corner frequency c is given by the following equation:
As can be seen from the equation, the corner frequency can be reduced by increasing either the inductance or capacitance or both. Typically, c is set to be 1/10th of the switching frequency of the converter to obtain a good attenuation.

Although it is easy to choose a filter corner frequency to reduce ripple effectively at the converter switching frequency, it is less easy to predict the attenuation of noise spikes which comprise a whole spectrum of harmonic frequencies. This is because at a certain frequency when the value of ZL and ZC become equal, the LC network may start to ‘resonate’ and noise can be amplified rather than attenuated. Above resonance, although there is still some noise attenuation, other parasitic effects begin to occur.

For example, the self-capacitance of the inductor produces another resonance peak, at a much higher frequency. This capacitance also tends to allow noise to ‘bypass’ the inductor. At higher frequencies, core losses in the inductor increase, and the AC resistance of the inductor wire increases because of the ‘skin effect’; moreover, the capacitor begins to act as a resistor as its impedance becomes small compared with its equivalent series resistance (ESR).

Fig. 2: An external filter with parasitic elements added
Capacitor equivalent series inductance (ESL) also produces high-frequency effects. If these parasitic elements are included, the equivalent circuit of the simple LC filter shown in Figure 1 becomes more like that shown in Figure 2 in reality.

4. Parasitic effects in inductors change noise attenuation performance

Using LLOSS 1 and LLOSS 2 along with RLOSS 1 and RLOSS 2 is a simplistic way to include the effect of frequency-dependent core losses in the circuit – different values of LLOSS give different impedances that allow different resistive elements RLOSS 1 and RLOSS 2 to have an effect at different frequencies.

More LLOSS/RLOSS networks can be added to make the model more accurate, but component values are difficult to compute from inductor data sheet information. Thus, for a complete model for a particular inductor and core, values must be found empirically.

Figure 3 shows ...

Want to read the whole Whitepaper?

ダウンロード/ログイン
アプリケーション